ما هو معامل K لآلة الثني وكيفية حسابه

ما هو عامل K لـ Press Brake؟

ال مكابح الضغط عامل K، المعروف أيضًا باسم عامل بدل الانحناء، هو معلمة بلا أبعاد تستخدم في ثني الصفائح المعدنية لتحديد مقدار استطالة المادة التي تحدث أثناء عملية الانحناء. يساعد في حساب بدل الانحناء، وهو الطول الإضافي للمادة المطلوب للثني.

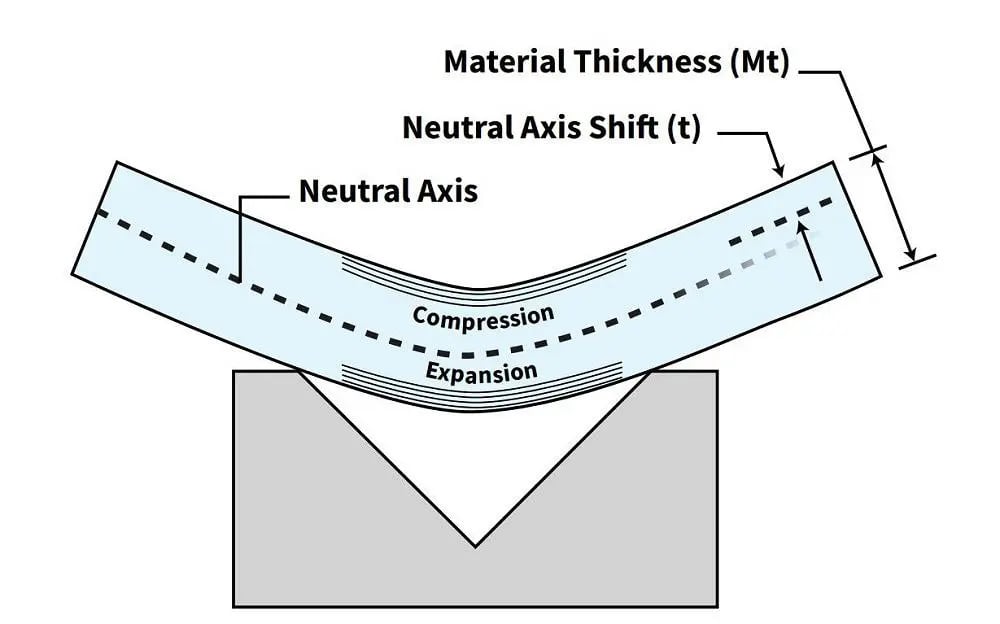
يأخذ عامل K في الاعتبار سمك المادة ونصف قطر الانحناء وخصائص المادة المراد ثنيها. وهو يمثل في الأساس النسبة بين المسافة من نصف قطر الانحناء الداخلي إلى المحور المحايد للمادة وسمك المادة.

يشير عامل K المنخفض إلى تمدد أقل للمواد أثناء الانحناء، بينما يشير عامل K المرتفع إلى تمدد أكبر. يعد عامل K ضروريًا للتنبؤ بدقة بزاوية الانحناء النهائية وتحقيق أبعاد انحناء دقيقة في تصنيع الصفائح المعدنية.

غالبًا ما يتطلب تحديد عامل K الصحيح لمادة معينة وإعداد الانحناء إجراء التجارب أو الرجوع إلى مواصفات المواد. يمكن أن يختلف بناءً على عوامل مثل نوع المادة والسمك والأدوات وطريقة الانحناء. بمجرد تحديد عامل K، يتم استخدامه عادةً في صيغ بدل الانحناء لحساب مقدار استطالة المادة وتحقيق أبعاد انحناء دقيقة.

ثني قطعة الاختبار

الهندسة العكسية لعامل k لـ مكابح الضغط الطريقة الوحيدة لتحديد قيمتها الفعلية، أو على الأقل أقرب ما يمكن إلى القيمة المثالية. يمكنك القيام بذلك من خلال تشغيل انحناءات الاختبار وقياس النتائج واستخراج عامل k من صيغة BA التي تتضمن النتائج التي قمت بقياسها. قد يكون هذا هو الخيار الأفضل لك، خاصةً إذا كنت تقوم بإنشاء جدول.

ولكن -وهذه نقطة مهمة- عليك أيضًا مراعاة تحمُّلات المواد، بما في ذلك الشد والخضوع والسمك. قد ينتهي بك الأمر إلى الحصول على بعض بيانات معامل k الدقيقة للغاية من قطعة اختبار، ولكن مادة قطعة الاختبار قد لا تتطابق مع خصائص المادة التي تثنيها في الإنتاج. بغض النظر عن ذلك، إذا وجدت للتو معامل BA عن طريق ثني قطع الاختبار، فقد لا تحتاج إلى معامل k على أي حال.

حساب عامل K، لا يتطلب قطعة اختبار

توجد طريقة أخرى لحساب عامل k دون ثني أي قطعة اختبار. إنها ليست طريقة مثالية، ولكن ثني قطعة اختبار ليس كذلك. لا يمكن أن تتغير خصائص المواد فحسب، بل يمكن أن تتغير أيضًا الخصائص الدقيقة للأدوات التي تستخدمها (مقادير مختلفة من الاحتكاك) وطرق التشكيل المختلفة.

رسم توضيحي يوضح عامل k في ثني الصفائح المعدنية

الشكل 1. العامل k، المعبر عنه بـ t/Mt، هو نسبة تصف تحول المحور المحايد إلى الداخل أثناء الانحناء.

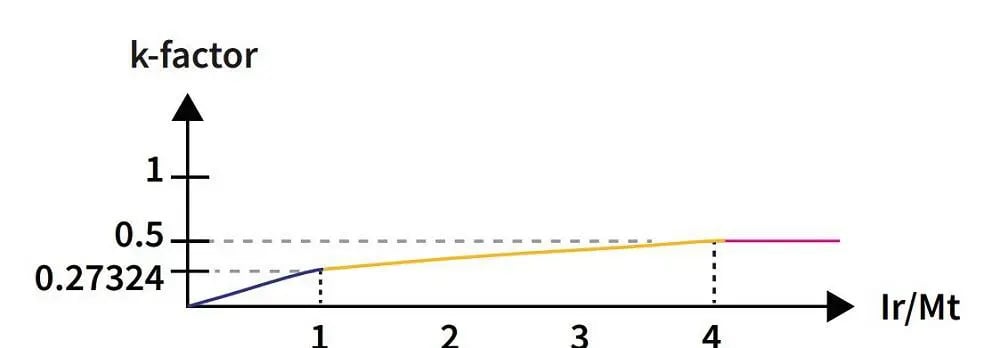
وبناءً على كل ما سبق، يمكنك البدء برسم معامل k بيانيًا، كما هو موضح في الشكل 3. يوضح هذا الحد الأقصى لمعامل k عند 50% من سمك المادة، كما هو موضح بالخط الأحمر. يمثل المكان الذي يلتقي فيه الخطان الأصفر والأحمر (النقطة 4 في الرسم البياني) نصف قطر انحناء داخلي يساوي أربعة أضعاف سمك المادة. وفوق ذلك، ستحسب عوامل أكبر من 50%، ولكن لا يجب عليك استخدامها. وكما يوضح الرسم البياني، تحتاج إلى الحفاظ على معامل k عند تلك القيمة القصوى 0.50.

الموقع 1 على الرسم البياني، حيث يلتقي الخطان الأزرق والأصفر، هو قيمة عامل k لنصف قطر داخلي حاد أو أدنى قابل للإنتاج لشكل الهواء. أي قيمة أقل من هذا تضع الانحناء في منطقة تتجاوز الحدود الفيزيائية للانضغاط - على الأقل بالنسبة لمادة الفولاذ الصلب الأساسية لدينا. وكما ذكرت مرات عديدة من قبل، فإن الانحناءات الحادة هي لعنة دقة تشكيل الهواء.

يمكن التعبير عن الحد الأدنى لعامل k لتكوين الهواء على أنه (4-π)/π، أو 0.27324. نطرح هذه القيمة من الحد الأقصى لعامل k وهو 0.5:

0.5 - 0.27324 = 0.22676

تعطينا هذه النتيجة نطاق العوامل المحتملة k كما هو موضح على الخط الأصفر في الرسم البياني. بعد ذلك، نقسم 0.22676 على 3:

0.2267/3 = 0.07558

هذا يعطينا المضاعف - أي الرقم الذي نضربه في نسبة نصف قطر الانحناء الداخلي إلى سمك المادة. نجد هذه النسبة عن طريق قسمة نصف قطر الانحناء الداخلي على سمك المادة. سأستخدم نصف قطر انحناء داخلي يبلغ 0.093 بوصة في مادة سمكها 0.062 بوصة.

0.093/0.062 = 1.5

ثم نضرب في المضاعف الخاص بنا، 0.07558، ونضيف النتيجة إلى عامل k الأدنى وهو 0.273:

0.07558 × 1.5 = 0.113

رسم توضيحي يوضح عامل k والتراجع الخارجي في ثني المعدن
الشكل 2. يصف عامل k تحول المحور المحايد إلى الداخل أثناء الانحناء. يتسبب هذا التحول في استطالة المعدن، وهو ما نستوعبه في حسابات الانحناء.

0.113 + 0.273 = 0.386

وهذا يجعل عامل k الخاص بنا يساوي 0.386. ويمكن تلخيص كل هذا في الصيغة التالية. مرة أخرى، يمكنك استنباط الحد الأدنى لعامل k والمضاعف من الرسم البياني في الشكل 2.

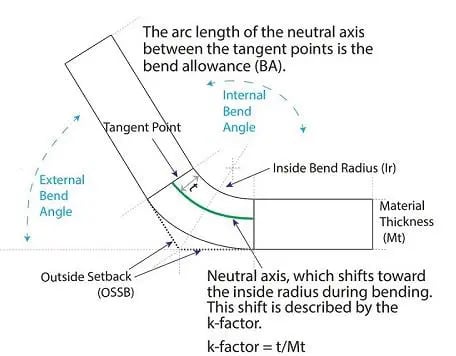
عامل K = [المضاعف × (نصف القطر الداخلي/سمك المادة)] + الحد الأدنى لعامل K

كسر بدل الانحناء

لقد وجدت عامل k. والآن ماذا نفعل بهذا الرقم؟ كيف يتم تطبيقه، ولماذا يجب أن تهتم؟ يعتمد سبب اهتمامك على جودة المنتج الذي تريد إنتاجه. إن فهم عامل k يحدث فرقًا كبيرًا. أولاً، ألق نظرة على صيغة BA الخاصة بك:

BA = [(0.017453 × نصف قطر الانحناء الداخلي) + (0.0078 × سمك المادة)] × زاوية الانحناء الخارجية

في هذه المعادلة، 0.017453 هو π/180، والذي يحول الدرجات إلى راديان، أو وحدات قياس للزوايا بناءً على نصف قطر الدائرة. نقوم بهذا التحويل لأن الدوال المثلثية في الحسابات الرياضية تتطلب عادةً أن تكون الزوايا بالراديان وليس بالدرجات. عندما تضرب الزاوية المحولة (بالراديان) في نصف القطر، فأنت تحسب في الأساس الطول على طول قوس الدائرة التي تشكلها الانحناءة. لذا، فإن π/180 مضروبًا في نصف القطر الداخلي يمثل طول القوس على طول المحور المحايد لدرجة واحدة من الزاوية.

يبدأ الجزء الثاني من المعادلة مرة أخرى بتحويل الدرجات إلى راديان (π/180)، ثم يتم ضربها بعامل k 0.4468، مما يعطينا الرقم 0.0078 في الصيغة. يمثل هذا التعويض الذي يحدث عندما يتحرك المحور المحايد إلى الداخل أثناء الانحناء، مما يتسبب في استطالة المادة وإضافة طول إلى أبعاد القطعة.

نحن نعلم الآن الطول الإجمالي للقوس والطول الإضافي الناتج عن تحول المحور المحايد. ومع ذلك، حتى الآن، كنا نحسب درجة واحدة فقط من زاوية الانحناء. الآن، نضرب زاوية الانحناء الخارجية الإجمالية، كما تم قياسها من خارج الانحناء. (ملاحظة: لا تستخدم أبدًا زاوية الانحناء الداخلية عند حساب زاوية الانحناء).

عامل واحد من بين العديد

لاحظ أن العديد من المتغيرات قد تؤثر على قيم معامل k، وخاصة إذا اختار المشغلون فتحات مختلفة للقالب أو استخدموا طرقًا مختلفة للتشكيل. لذا، ما هي "أفضل" طريقة لإعادة حساب جداول معامل k؟ ربما تكون جدول بيانات Excel. ربما يمكنك ثني قطع الاختبار. فقط اعلم أن معامل k ليس سوى متغير واحد من بين العديد من المتغيرات التي يجب مراعاتها.